Previous Page | Right click this page to print.
Pearson's Square and Double Pearson's Square
Next, we are going to cover some simple hand calculation techniques for formulating very simple rations. These two techniques are called Pearson’s Square.
In our first example, we are going to formulate a finishing diet for a pen of beef animals that contains 80% TDN on a dry-matter basis.

We are going to use two feeds, a roughage which contains 60% TDN on a dry-matter basis and a concentrate mix containing 83% TDN on a dry-matter basis. So with the Pearson’s Square, we write down the feedstuff, so roughage, below that we would write down concentrate. Beside each of those we would write down the TDN concentration, 60% for roughage and 83% for concentrate. And to the right and the middle of those two numbers, we would write our goal, in this case it is 80% TDN. We subtract down the diagonals and use absolute numbers. So 60 minus 80 equals 20, or the absolute value is 20, 83 minus 80 equals 3. Now at this point, these numbers are in parts. We need to sum those, 3 plus 20 equals 23. If we divide 3 and 20 by the total, which is 23, we will get the percent of diet that is roughage and is concentrate. So, 3 divided by 23 is 13% and 20 divided by 23 is 87%. Now the way you read the Pearson’s Square is straight across. If you look at roughage it is 13% of the diet and concentrate is 87% of the diet.
Now suppose our roughage is a silage and contains 35% dry-matter and our concentrate contains 91% dry-matter.
% of
Diet DM |
% DM
|
Parts
|
% of
Diet AF |
||
|---|---|---|---|---|---|
Roughage |
13.0 |
35 |
37.3 |
28.1 |
=13/(35/100)=37.3/132.8 |
Concentrate |
87.0 |
91 |
95.6 |
71.9 |
=87/(91/100)=95.6/132.8 |
132.8 |
100.0 |
||||
And we want to know what the ingredient composition is on an as-fed basis. Now when we are formulating diets for a certain composition, it is always on a dry-matter basis. But when we are delivering feed to animals, it is always on an as-fed basis. And so we have to do some conversions after the balancing is done, so that we can give this to the feeder, so that they deliver the right amount of feed to the animals. Again our roughage is 13% of the diet and our concentrate is 87% of the diet on a dry-matter basis. The roughage contains 35% dry-matter and the concentrate 91%. If we convert the dry-matter percentages to a decimal form, so for the roughage it would be 35 divided by 100 so that is .35. Thirteen (13) divided by .35 equals 37.3. And again this is parts, it doesn’t mean anything at this point. For our concentrate, we would divide 87% of the diet dry-matter divided by its dry-matter percent, which is .91, and that would give us 95.6 parts. If we sum these two, we get 132.8. To convert these parts to percentages, would be 37.3 divided by 132.8, so 28.1% of the diet on an as-fed basis is roughage. Ninety-five point six (95.6) divided by 132.8 would give us 71.9% of the diet on an as-fed basis is concentrate. When we are formulating the diet at the farm to deliver to these animals, the percentages would be 28.1% roughage and 71.9% concentrate.
Now suppose we want to calculate the cost per ton.
% of
Diet AF |
$/T
AF |
$ |
||
|---|---|---|---|---|
Roughage |
28.1 |
35 |
9.82 |
=28.16/100*35 |
Concentrate |
71.9 |
130 |
93.53 |
=71.9/100*130 |
103.35 |
||||
Suppose the roughage costs $35 per ton and the concentrate costs $130 per ton. Remember, prices are quoted on an as-fed basis. The roughage is 28.1% of the diet and the concentrate is 71.9% of the diet on an as-fed basis. Our costs are $35 and $130, respectively for roughage and concentrate. Per ton of diet, the roughage is contributing $9.82. We arrived at that by dividing 28.1 by 100 and times it by the price, which is $35. The concentrate is contributing 93.53 or $93.53 per ton. The calculation was 71.9 divided by 100 times the price, which is $130. If we sum those two numbers, the cost per ton of our ration is $103.35.
Suppose the steers, in this example, are consuming 25 lbs of as-fed feed per day and we want to know what the cost is per animal per day.
$1.29 =25/2000*$103.35
So, this is a pretty easy calculation. We know how many lbs of as-fed feed they are consuming per day and we know the cost per ton on an as-fed basis. If we convert how much each animal is consuming to a ton basis or 25 lbs divided by 2,000 lbs per ton and we multiply that by the cost per ton which is $103.35, our cost per animal per day is $1.29.
We can go even further and look at some efficiency factors.
=25 AF lbs x 28.1% roughage = 7.0 AF lbs x 35% DM = 2.5 lbs DM
=25 AF lbs x 71.9% concentrate = 18.0 AF lbs x 91% DM = 16.4 lbs DM
Total of 18.9 lbs DM
Feed Efficiency = 5.4 =18.9 lbs DM/3.5 lbs gain
Cost per pound of gain = $ 0.37 =1.29/3.5
Suppose the steers are gaining 3.5 lbs per day and we want to know what our feed efficiency is or lbs of feed per lbs of gain. We will also want to know what the cost is per lb of gain. Now, if this was a relatively high dry-matter diet, or most of the feeds were 88-92% on a dry-matter basis, the next calculation would be very simple. But, we threw a wrinkle into it and assumed that our roughage was a silage or a wet feedstuff. We have to do some back-calculation to determine how many lbs of dry-matter the animals are consuming per day. If we start with what is know, the animals are consuming 25 lbs of as-fed feed per day. We know that 28.1% of this is roughage. So that means the animals are consuming 7 lbs of as-fed roughage per day. We know that the roughage is 35% dry-matter. So, 7 times .35 would give us 2.5 lbs of roughage dry-matter per day. For the concentrate, again the animals are consuming 25 lbs of as-fed feed per day and the concentrate makes up 71.9% of the diet. So the animals are consuming 18% of as-fed lbs of concentrate per day. Eighteen (18) times the dry-matter percent of concentrate or .91, would give us 16.4 lbs of dry-matter from the concentrate per day. So, 16.4 plus 2.5 would give us 18.9 lbs of dry-matter per day. If we get back to what we originally want to calculate, that is feed efficiency, lbs of feed per lb of gain. If the animals are consuming 18.9 lbs of dry-matter per day and gaining 3.5 lbs, our feed efficiency would be 5.4 lbs of feed per lb of gain. Our cost per lb of gain would simply be the cost per head per day which is $1.29, divided by 3.5 lbs of gain per day. Cost per lb of gain, in this example, would be 37 cents per lb.
So in the last example, we used two feedstuffs. The Double Pearson's Square is about as sophisticated as you can get in hand formulation. In our next example, we are going to go through a Double Pearson’s Square.
Corn Silage |
24.7 lbs |
(74.9/100 x 30/100 x 110) |
Concentrate |
18.1 lbs |
(17.9/100 x 92/100 x 110) |
Alfalfa Hay |
7.0 lbs |
(7.2/100 x 88/100 x 110) |
Total |
49.8 |
$5.42 =100/75*$4.07
What a Double Pearson’s Square allows us to do is use more than two feedstuffs and actually we can formulate a diet for three feedstuffs. Suppose we want a final mix with 16% crude protein and 72% TDN. We have three feedstuffs to choose from, corn silage, concentrate, and alfalfa hay. The corn silage is 9% crude protein and 66% TDN. The concentrate is 24% crude protein and 79% TDN. The alfalfa hay is 20% crude protein and 68% TDN. Okay, so we just select two feedstuffs and we can go in order. We will select corn silage and concentrate for our first Pearson’s Square.

The first nutrient that we are going to formulate for is crude protein. Corn silage is 9% crude protein and our concentrate is 24% crude protein. We put 16% crude protein, or our goal, in the center and subtract down the diagonals. Nine (9) minus 16 is 7 or the absolute value is 7. Twenty-four (24) minus 16 is 8. So in our first square, or mix 1, it is 8 parts corn silage and 7 parts concentrate. The sum of those is 15. If we divide 8 by 15 that equals 53.3% of the diet dry-matter as corn silage. And 7 divided by 15 is 46.7% of the diet dry-matter as concentrate. From our first square, we can also calculate the TDN of that square. Corn silage is 68% TDN times .533 would give us 36.3% TDN contribution from corn silage. Similarly, for concentrate it would be 79 times .467 which equals 36.9. Sum the contribution of TDN from mix 1, for corn silage and concentrate, 36.3 plus 36.9 gives us a total of 73.1% TDN. We have now formulated one square that contains 16% protein and 73.1% TDN. The feedstuff we didn’t use was alfalfa hay which is 20%. We can use either of the first two feedstuffs in the second square also. However, in order for the square to balance, we have to use corn silage because we must have one feedstuff with crude protein less than 16 and one feedstuff greater than 16. So corn silage must also be used in the second Pearson’s Square.

So in mix 2, we are going to use corn silage 9% protein and alfalfa hay 20% protein. Again, put 16% in the center. Subtract down the diagonal, 9 minus 16 equals 7. Subtract up the diagonal, 20 minus 16 equals 4. So, 4 parts corn silage, 7 parts alfalfa hay. If we sum those we get 11. If we divide 4 by 11 that gives us 36.4% of the diet in mix 2 is corn silage and 7 divided by 11 is 63.6% alfalfa hay. We can also calculate the TDN of the second square or mix 2. Sixty-eight (68) times .634 equals 24.7% TDN from corn silage and 68 times .636 is 43.3% TDN from alfalfa hay. Twenty-four point seven (24.7) plus 43.3 is 68% TDN. Now this example worked out nicely. So even though we are balanced for crude protein at this point, we are still not balanced for TDN. So our third square, will be mix 1 TDN and mix 2 TDN balancing for 72%.
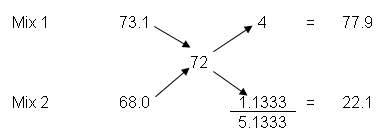
Now again, you have to have one TDN that is greater than 72 and one that is less than 72. And we have achieved that, mix 1 was 73.1% TDN and mix 2 was 68% TDN. If we subtract down the diagonal, 73.1 minus 72 equals 1.13. Subtracting up the diagonal, 68 minus 72 equals 4. So 4 parts mix 1 and 1.13 parts mix 2 or for a total of 5.13 parts. Four (4) divided by 5.13 equals 77.9% mix 1 and 1.13 divided by 5.13 equals 22% mix 2.
Finally, we are ready to calculate the ingredient composition.
Corn silage |
49.6 |
=(53.3/100*77.9)+(36.4/100*22.1) |
Concentrate |
36.4 |
=46.7/100*77.9 |
Alfalfa Hay |
14.0 |
=63.6/100*22.1 |
100.0 |
Now remember, corn silage was in two of the squares, both in mix 1 and mix 2. For corn silage, to figure out the total in the diet, it would be 53.3% from mix 1 times the percentage of mix 1 that came out of square 3 which was 77.9. Then, we add to that, the corn silage that came out of mix 2, so 36.4 divided by 100 times the percent of mix 2 in the final diet which is 22.1. So to recap that, it is 53.3 divided by 100 times 77.9 plus 36.4 divided by 100 times 22.1. Corn silage makes up 49.6% of the diet. The other two ingredients are simpler because they were only in one square. Concentrate, which was in mix 1, was 46.7% and mix 1 makes up 77.9% of the final diet, so 46.7 divided by 100 times 77.9 equals 36.4% of the diet is concentrate. Alfalfa hay which was in square 2 or mix 2, 63.6% divided by 100 times the percent mix 2 in the final square which was 22.1, so alfalfa makes up 14% of the diet.
Remember that this is on paper, this is our formulation, but what someone would deliver to the animals at the farm would probably be quite different. In this case, since we are using corn silage which is low in dry-matter, our as-fed diet will differ dramatically from our dry-matter diet.
% of Diet DM |
% DM |
Parts |
% of Diet AF |
||
|---|---|---|---|---|---|
| Corn Silage |
49.6 |
30 |
165.3 |
74.9 |
=49.6/(30/100)=165.3/220.8 |
Concentrate |
36.4 |
92 |
39.5 |
17.9 |
=36.4/(92/100)=39.5/220.8 |
| Alfalfa Hay |
14.0 |
88 |
16.0 |
7.2 |
=14.0/(88/100)=16.0/220.8 |
220.8 |
100.0 |
The dry-matter percent for corn silage is 30, for concentrate 92, and for alfalfa hay 88. First, we determine how many parts of each of the three ingredients will make up the as-fed diet. So 49.6% of the diet as dry-matter divided by the decimal form of the dry-matter concentration which is .3 equals 165.3 parts. For concentrate, 36.4 divided by .92 equals 39.5. And for alfalfa hay, 14 divided by .88 equals 16 parts. If we sum the parts, it equals 220.8. If we divide the parts of corn silage, concentrate and alfalfa hay by the total parts, we will get the percent of the diet on an as-fed basis. For corn silage, it would be 165.3 divided by 220.8 or corn silage would make up 74.9% of the as-fed diet. For concentrate, 39.5 divided by 220.8 would equal 17.9% of the as-fed diet as concentrate. And alfalfa hay, 16% divided by 220.8 would equal 7.2% of the as-fed diet.
Next, we want to calculate how much the diet costs us per ton as-fed.
| % of Diet AF |
$/T AF |
$ |
||
|---|---|---|---|---|
Corn Silage |
74.9 |
35 |
26.20 |
=74.9/100*35 |
Concentrate |
17.9 |
200 |
35.81 |
=17.9/100*200 |
| Alfalfa Hay |
7.2 |
165 |
11.93 |
=7.2/100*165 |
73.94 |
We know the percentages as-fed in the diets and our costs are $35 per ton for corn silage, $200 for concentrate, and $165 for alfalfa hay. Our contribution to the total cost for corn silage is, 74.9 divided by 100 times the cost which is $35, so $26.20. For concentrate, 17.9 divided by 100 which gives us .179 times the cost which is $200 equals $35.81. For alfalfa hay, 7.2 divided by 100 times $165 per ton which equals $11.93. The cost per ton of the diet we are delivering to the animals is $73.94.
Suppose the cows in this example are consuming 110 lbs of as-fed feed per day and we want to know what the cost is per animal per day or per head per day. If we convert what the animals are consuming to a ton basis, 110 lbs divided by 2,000 lbs per ton times our cost which is $73.94 would equal $4.07 per head per day.
We also want to know how many lbs of dry-matter the cows are consuming. Again, we have to back-calculate and determine how many lbs of dry-matter for each of the three ingredients the animals are consuming.
Corn Silage |
24.7 lbs |
(74.9/100) x (30/100 x 110) |
Concentrate |
18.1 lbs |
(17.9/100) x (92/100 x 110) |
Alfalfa Hay |
7.0 lbs |
(7.2/100) x (88/100 x 110) |
Total |
49.8 |
The things we do know are that the animals are consuming 110 lbs of dry-matter per day and of this 74.9% is corn silage, 17.9% is concentrate and 7.2% is alfalfa hay. If we divide 74.9 by 100 and multiply that by the dry-matter percent for corn silage and then multiply that by the total lbs as-fed, we will get how many lbs of dry-matter coming from corn silage. The calculation would be 74.9 divided by 100 times 30 divided by 100 times 110 lbs of feed per day gives us 24.7 lbs of corn silage per day. For concentrate, it would be 17.9 divided by 100 times the dry-matter concentration which is 92 divided by 100 times the total amount of feed consumed per day which is 110 and that would equal 18.1 lbs of dry-matter from concentrate per day. For the alfalfa hay, it is 7.2 divided by 100 times 88 divided by 100 times 110 lbs of feed per day which would equal 7 lbs of alfalfa hay. So if we sum those, it is 49.8 lbs of dry-matter per day that the cows are consuming.
And our last calculation is, what is the cost per 100 lbs of milk produced
per day? If these cows were producing 100 lbs of milk, the cost per 100
weight and the cost per head per day would be the same, $4.07. However,
in this example our cows are producing 75 lbs of milk per day, so would
it cost more or less to produce 100 lbs of milk? Well, it would cost more
because our cows are producing less than 100 lbs of milk. The calculation
would be 100 lbs, which is our basis per 100 lbs of milk, divided by how
many lbs of milk the cows are producing. In this example, it is 75 lbs
of milk. And that is multiplied by the cost per animal per day which is
$4.07. On this farm, it is costing us $5.42 to produce 100 lbs of milk.
Previous Page | Right click this page to print.