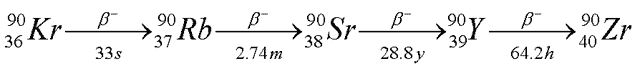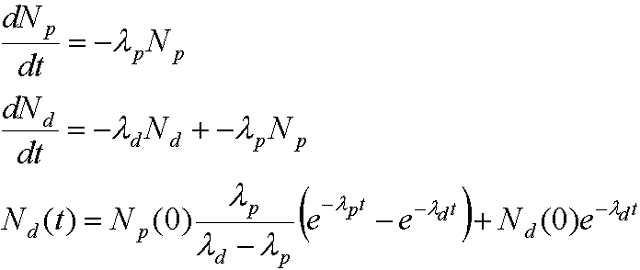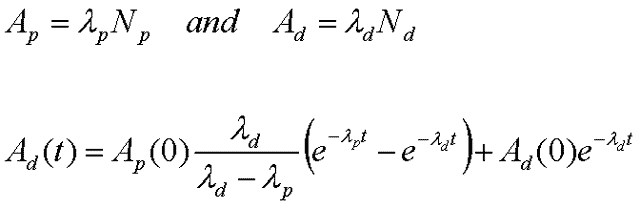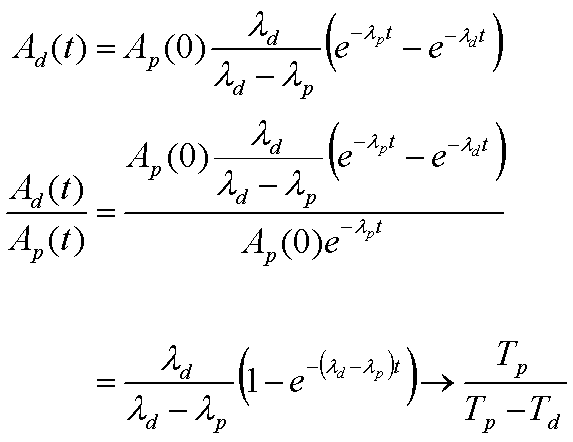Topic 4 - Sources of Radiation

Decay Chains

Serial Transformation

• Radioactive decay chains
• Described by Bateman equations
  • Secular
  • Transient
  • No equilbrium
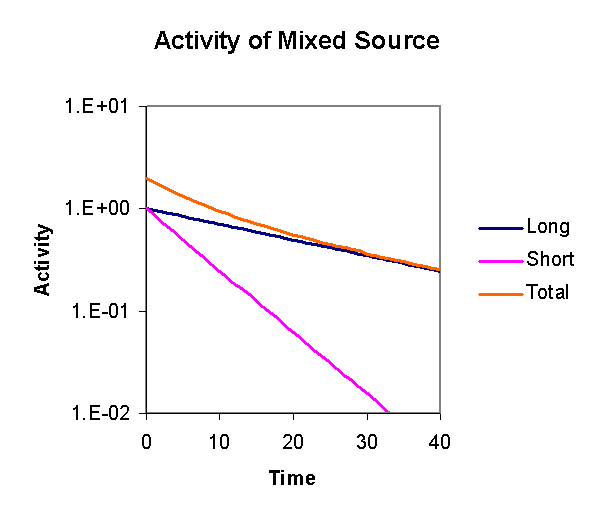
• Mixtures of unrelated nuclides

Decay schemes

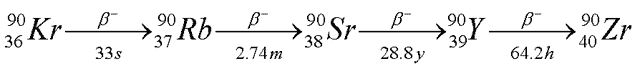
• Fission fragment example
  • ⁹⁰Kr rapidly disappears
  • ⁹⁰Rb created and also disappears
  • ⁹⁰Sr present for extended period, while ⁹⁰Y grows in
• Final product is stable

Decay Equations

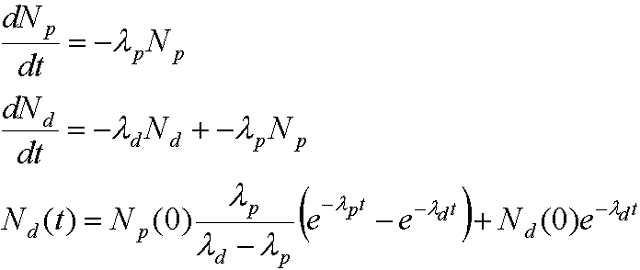
• Where
  • N_p is the # of atoms of parent
  • N_d is the # atoms of daughter
  • l_{p or d} is the decay constant (ln2/T_½ ) of parent or daughter

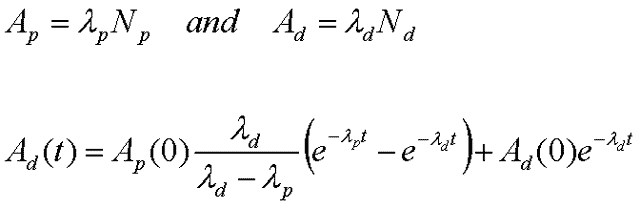
• The second term in the activity equation is just the residual daughter product activity remaining from any that was present at t=0.
• This equation is simpler if we set this value to zero and just examine the first term. The equation for activity of the daughter is known as the Bateman equation

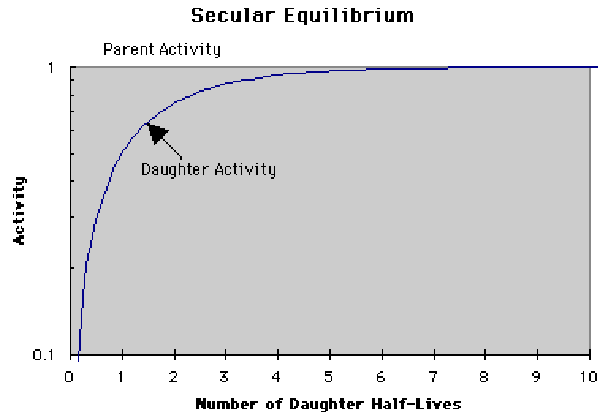
Secular Equilibrium

• Half-life of parent is >>> daughter,
• Decrease of parent is negligible over observation period
  • Example: ²²⁶Ra (half-life =1620 yr) decays to ²²⁶Rn (half-life 4.8 days).
  • The observation period is so small relative to 1620 years that 1 gram of Ra-226 was used to define the unit of radioactivity - the Curie.
• When the half-life of parent is >>the daughter, the Bateman Equation reduces to:

Secular Equilibrium

• The plot of parent and daughter activity in secular equilibrium is:

Transient Equilibrium

• When the parent half-life is of the order of the observation time and the daughter half-life is considerably shorter (but not negligibly shorter).
• Examples:
  • ¹³²Te (78 hours) decaying to ¹³²I (2.3 hours)
  • ¹¹³Sn decaying to ^113mIn (1.7 hours)
  • ⁹⁹Mo parent - ^99mTc
    
    

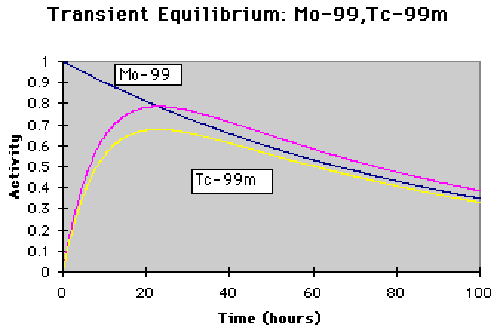
• Upper curve for ^99mTc is the straight application of the Bateman equation.
• Lower curve: takes into account the fact that about 10% of the ⁹⁹Mo decays go promptly to ⁹⁹Tc
• Ratio of parent to daughter

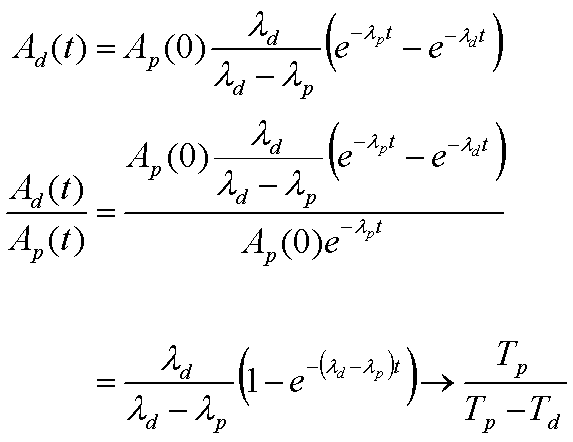
Maximum Activity

•  Use calculus to find the time at which the daughter reaches a maximum

No equilibrium

• When the daughter half-life is longer than the parent half-life, there is no equilibrium established between them. As the short-lived parent dies off, the activity of the daughter starts from zero, grows to a maximum, then falls slowly at its own decay rate (the parent having since died off and not able to influence daughter rate any further).

Mixed Sources